What are Integers?

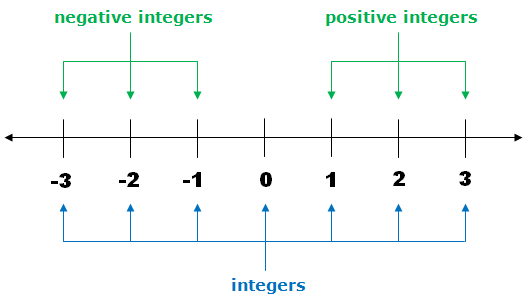
The number system consists of real, natural, whole, fractional, integer, rational, irrational, and complex numbers. A number that can be written without a fractional part is known as an integer. In other words, these are numbers that consist of positive whole numbers or counting numbers, zero and negative numbers. Thus, integers do not consist of fractions. We can perform arithmetic operations on integers, and they follow certain properties. In this article, we learn more about integers and associated concepts.

Properties of Integers

1. Closure Property

When two integers are added or multiplied, it gives an integer thus, indicating that the closure property holds. If p and q are integers then, p + q = integer and p * q = integer.

2. Commutative Property

If two integers are added or multiplied if we interchange the placement of the numbers, the result will not get affected. Thus, p + q = q + p and p * q = q * p. This property does not hold for division and subtraction.

3. Associative Property

If two integers are added or multiplied together, the order of performing this operation will not change the result. (p + q) + r = p + (q + r) and (p * q) * r = p * (q * r).

4. Distributive property

According to this property, multiplication distributes over addition and vice versa for integers. Thus, it can be expressed as (p + q) * r = pr + qr and (p * q) + r = (p + r) * (q + r).

5. Identity Property

When we add 0 to an integer we get the integer itself; p + 0 = p.

When we multiply an integer with 1, the product is the integer itself; p * 1 = p.

Rules of Arithmetic Operations

• When two positive integers are added together, the sum is a positive integer; E.g., 4 + 10 = 14.
• When two negative integers are added together, the sum is a negative integer; E.g., -19 – 11 = – 30.
• If we subtract a positive integer that is greater in value from another smaller positive integer, the difference will result in a positive number. E.g., 45 – 10 = 35.
• If we add a positive integer that is smaller in value to a negative integer, then the result will be the difference between the two numbers with a negative sign. E.g., – 89 + 4 = -85.
• When two positive integers are multiplied, their product is a positive integer. For example, 4 * 5 = 20.
• If two negative integers are multiplied, their product is a positive integer. For example, (-5) * (- 4) = 20.
• If one negative and one positive integer are multiplied together, then their product is a negative integer. E.g., 5 * (- 4) = -20.
• The sum of any integer and its corresponding negative part is always 0. Thus, 7 + (-7) = 0.
• If we multiply any integer with its reciprocal, the product equals 1. E.g., 8 * (⅛) = 1.

Applications of Integers

Integers have widespread use in real life. They are usually used to represent contradicting situations. It is used to represent temperatures above and below zero, giving people an idea of how the weather is. It helps to quantify the hotness and coldness of the climate. Such a method is very useful for scientists who study biodiversity and habitat as well as the collective health of the earth.

Conclusion

As we can see, the study of integers also involves other concepts such as whole numbers. Thus, to build a strong foundation, kids need to turn to Cuemath which is an online educational platform, to improve their understanding of the topic. At Cuemath, the certified tutors help kids maintain their own pace of learning while ensuring that they have an enjoyable learning experience